Лако-красочные материалы — производство

A sketch of a polymer brush is shown in Fig. 1. We call a the graft density or the number of grafted chains per unit area of the surface. Each chain has N monomers. In a dilute solution the chain radius of gyration, R, is R ~ N1/2a for Gaussian chains in a в solvent (a is the monomer size) and R ~ Nva in a good solvent with a swelling exponent v = 3/5. If the surface density is small (aR2 ^ 1) different chains on the surface do not see each other and behave as independent chains of size roughly equal to R on the surface. This is called

н

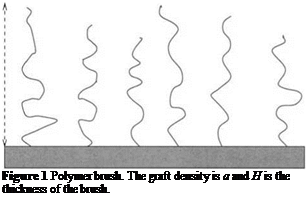the mushroom regime. If the surface density becomes larger, aR2 > 1, neighboring chains interact and repel each other in a good solvent. The chains stretch in the direction per­pendicular to the grafting surface. In order to calculate the thickness of the polymer brush, we use a Flory type of argument. We assume that all the chain end points are located on the outside of the layer and thus all the chains have the same end-to-end distance H equal to the thickness of the brush. In a first approximation, we consider that the monomer concentration inside the grafted layer is constant and equal to c = aN/H. The free energy of the chains is the sum of the Gaussian elastic free energy and the repulsive excluded volume interaction energy between the chains. The Gaussian elasticity measures the entropy cost due to the chain stretching. Polymer chains behave as springs and the elastic energy per unit area reads Fel = (3/2) akT(H2/Na2) where T is temperature and k the Boltzmann constant. The interaction energy per unit area can be obtained from a virial expansion Fint = (1/2) vc2HkT where v is the positive second virial coefficient between monomers (the so-called excluded volume parameter); it is positive in a good solvent. The minimization of the total free energy Fel + Fint gives the equilibrium thickness [13]

H — N(aa2)1/3v1/3 (1)

The thickness increases linearly with molecular weight and the polymer chains in a grafted layer are strongly stretched; this is consistent with the large thicknesses measured experimentally. The free energy per chain д is proportional to NkTa2/3; it gives the energy barrier to insertion of one extra chain into the brush. This energy is much larger than kT and the rate of grafting of the chain must be very low in any experiment where the grafted layer is built up by adsorption from a dilute solution.

This Alexander-de Gennes model of polymer brushes gives a scaling description in good agreement with experimental results for the thickness of grafted polymer layers [14]. However, two of the starting points—the facts that the monomer concentration is constant over the layer and that the end points are all at the surface of the layer—are not consistent with experiments. These two constraints are relaxed in the more refined self-consistent field model proposed independently by Milner et al. [15] and Zhulina et al. [16]. The idea of this model is to consider in a mean field approach the conformation of one chain in an effective potential U(z) = kTvc(z) where c(z) is the local concentration at a distance z from the grafting surface. The potential is then calculated self-consistently to satisfy all the constraints of the problem using an elegant analogy to classical mechanics. The monomer concentration is found to decay parabolically from the grafting
surface: vc(z) = (Зп2/8N2a2)(H2 — z2). The other important result is that the chain end points are distributed throughout the layer. The density of end points vanishes on the grafting surfaces, it increases to a maximum at about three-quarters of the way into the layer and then decreases back to zero. These results are in good agreement with neutron scattering experiments [14].

When the grafted layer is built by physical adsorption of the chain end points on the surface either one or both end points may adsorb. If only one end point adsorbs, a standard grafted layer is formed and the end point graft energy monitors the chain graft density. If the two end points adsorb, a mixture between chains grafted by one end and chains grafted by both ends (loops) is obtained on the surface [17]. Within the self-consistent field approach a chain grafted by both ends can be cut at its midpoint and is thus equivalent to two grafted chains each containing N/2 monomers. The grafted layer can be considered as a polydisperse layer of chains of N and N/2 monomers. The fraction of chains grafted by both ends increases with the graft density E of the end points from zero if E/д < 1 to one if E/д > 1 where д is the chemical potential of one chain in the brush (the energy per chain).